The squirmer is a model for a spherical microswimmer swimming in Stokes flow. The squirmer model was introduced by James Lighthill in 1952 and refined and used to model Paramecium by John Blake in 1971.[1] [2] Blake used the squirmer model to describe the flow generated by a carpet of beating short filaments called cilia on the surface of Paramecium. Today, the squirmer is a standard model for the study of self-propelled particles, such as Janus particles, in Stokes flow.[3]
Velocity field in particle frame
Here we give the flow field of a squirmer in the case of a non-deformable axisymmetric spherical squirmer (radius ).[1][2] These expressions are given in a spherical coordinate system.
Here are constant coefficients, are Legendre polynomials, and .
One finds .
The expressions above are in the frame of the moving particle. At the interface one finds and .
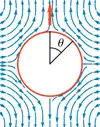
 Shaker, | 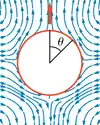 Pusher, |  Neutral, |  Puller, | 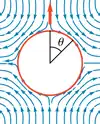 Shaker, |  Passive particle |
 Shaker, | 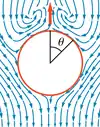 Pusher, | 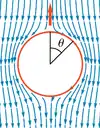 Neutral, | 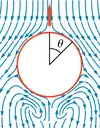 Puller, |  Shaker, | 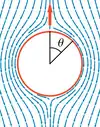 Passive particle |
| Velocity field of squirmer and passive particle (top row: lab frame, bottom row: swimmer frame, ). | |||||
Swimming speed and lab frame
By using the Lorentz Reciprocal Theorem, one finds the velocity vector of the particle . The flow in a fixed lab frame is given by :
with swimming speed . Note, that and .
Structure of the flow and squirmer parameter
The series above are often truncated at in the study of far field flow, . Within that approximation, , with squirmer parameter . The first mode characterizes a hydrodynamic source dipole with decay (and with that the swimming speed ). The second mode corresponds to a hydrodynamic stresslet or force dipole with decay .[4] Thus, gives the ratio of both contributions and the direction of the force dipole. is used to categorize microswimmers into pushers, pullers and neutral swimmers.[5]
| Swimmer Type | pusher | neutral swimmer | puller | shaker | passive particle |
| Squirmer Parameter | |||||
| Decay of Velocity Far Field | |||||
| Biological Example | E.Coli | Paramecium | Chlamydomonas reinhardtii |
The above figures show the velocity field in the lab frame and in the particle-fixed frame. The hydrodynamic dipole and quadrupole fields of the squirmer model result from surface stresses, due to beating cilia on bacteria, or chemical reactions or thermal non-equilibrium on Janus particles. The squirmer is force-free. On the contrary, the velocity field of the passive particle results from an external force, its far-field corresponds to a "stokeslet" or hydrodynamic monopole. A force-free passive particle doesn't move and doesn't create any flow field.
See also
References
- 1 2 Lighthill, M. J. (1952). "On the squirming motion of nearly spherical deformable bodies through liquids at very small reynolds numbers". Communications on Pure and Applied Mathematics. 5 (2): 109–118. doi:10.1002/cpa.3160050201. ISSN 0010-3640.
- 1 2 Blake, J. R. (1971). "A spherical envelope approach to ciliary propulsion". Journal of Fluid Mechanics. 46 (1): 199–208. Bibcode:1971JFM....46..199B. doi:10.1017/S002211207100048X. ISSN 0022-1120. S2CID 122519123.
- ↑ Bickel, Thomas; Majee, Arghya; Würger, Alois (2013). "Flow pattern in the vicinity of self-propelling hot Janus particles". Physical Review E. 88 (1): 012301. arXiv:1401.7311. Bibcode:2013PhRvE..88a2301B. doi:10.1103/PhysRevE.88.012301. ISSN 1539-3755. PMID 23944457. S2CID 36558271.
- ↑ Happel, John; Brenner, Howard (1981). Low Reynolds number hydrodynamics. Mechanics of fluids and transport processes. Vol. 1. doi:10.1007/978-94-009-8352-6. ISBN 978-90-247-2877-0. ISSN 0921-3805.
- ↑ Downton, Matthew T; Stark, Holger (2009). "Simulation of a model microswimmer". Journal of Physics: Condensed Matter. 21 (20): 204101. Bibcode:2009JPCM...21t4101D. doi:10.1088/0953-8984/21/20/204101. ISSN 0953-8984. PMID 21825510. S2CID 35850530.